The Waves app consists of a collection of five different simulations that deal with traveling waves, standing waves, and beats. There are two different traveling wave simulations, one for transverse waves and another for longitudinal waves. There are also two simulations for standing waves, one for transverse waves and one for longitudinal waves.
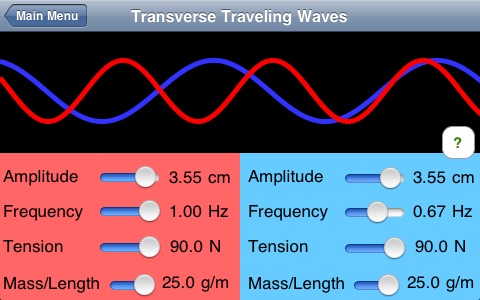
Simulation 1 - Transverse Traveling Waves. Two independent traveling waves are shown. You can adjust the amplitude and the frequency of each of the waves individually. This simulates the motion of waves traveling along strings, so you can also investigate how adjusting the tension applied to a string and/or the weight of the string (via the mass per unit length) affects the wave on that string.
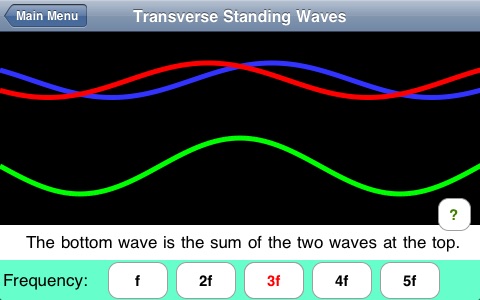
Simulation 2 - Transverse Standing Waves. This is a model of standing waves on a string that is fixed (in other words, held in place) at both ends. An example of such a string would be a guitar string or a piano string. Using the buttons, you can choose between showing the fundamental (lowest frequency) standing wave, or showing the second, third, fourth, or fifth harmonics of the fundamental. The frequencies of the harmonics are integer multiples of the frequency of the fundamental. Note that the standing wave is a superposition (sum) of two identical traveling waves, one moving to the right and one moving to the left. Because the string is fixed at both ends, the standing waves must have nodes (points of zero motion) at the ends.
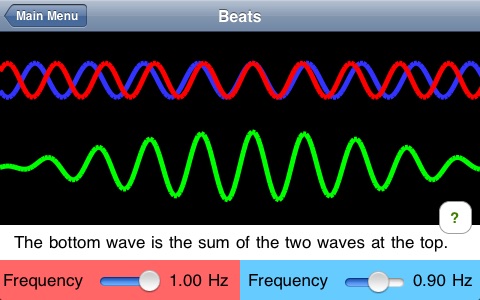
Simulation 3 - Beats. The phenomenon of beats is another example of superposition. In this case, the two waves have slightly different frequencies, so they gradually drift from being completely in phase with one another (where the resulting wave has a large amplitude) to being completely out of phase with one another (where the resulting wave has a small amplitude) to being back in phase again. The rising and falling of the loudness of the wave is what we refer to as beats - the beat frequency is equal to the frequency difference between the two individual waves.
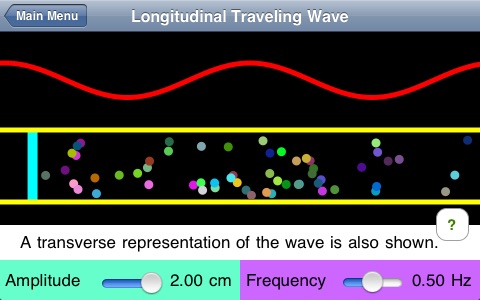
Simulation 4 - Longitudinal traveling wave. This is a simulation of how sound waves propagate through air. The top part of the screen shows a transverse representation of the traveling wave, with the longitudinal representation below that. The longitudinal wave is shown passing through a pipe, but the pipe is not necessary. The motion of the dots represents the average motion of all the air molecules that are in the vicinity of the dots. Note that there is no net flow of air molecules in any direction - the molecules oscillate about an equilibrium position, on average, to transfer the energy of the wave through the air.
Simulation 5 - Longitudinal standing waves. This simulation shows some of the various standing waves that can occur in a pipe - this is a model of what happens in wind instruments such as organ pipes, flutes, etc. As with simulation 4, the top of the screen shows a transverse representation of the standing wave, with the longitudinal representation shown below that. The left end of the pipe is always open in this simulation, and you can choose between leaving the right end open or closing it. At an open end, the air molecules are free to move, and the standing wave has an anti-node (a point of maximum displacement from equilibrium) for molecule displacement. At a closed end, the molecules are not free to move, so the standing wave has a node for molecule displacement (that is, the molecules dont move there). This leads to different resonance conditions for the pipe, so the frequencies that produce standing waves in an open pipe are different from the frequencies that give standing waves when the pipe has a closed end.